In this series of posts on optical rotations, I firstly noted Kirkwood’s 1937 attempt to correlate the optical rotation of butan-2-ol with its absolute configuration. He had identified as essential knowing the relative orientation (the term conformation was not yet in common use) of the two methyl groups (the modern terms are gauche vs anti) and also that of the hydroxyl group, noting that anisotropy from this group could influence his result (he had assumed it was linear, or axially symmetric). I then looked at D-(+)-glyceraldehyde, noting that this species itself has a strongly negative rotation and that it is the hydrated diol that results in a positive rotation and hence the (+) designation. Here I take another look at this latter system to see what effect adding explicit water molecules to the unhydrated form of glyceraldehyde might have on its computed rotation, on the premise that strong hydrogen bonds can also contribute anisotropy to the system.
Firstly, here again are the computed results for glyceraldehyde on its own, albeit encased in a continuum solvent field for water (SCRF=water). At 303K and 589nm, the computed rotation is -193° compared to -147° inferred from the population of the aldehyde.[cite]10.1135/cccc19841167[/cite]
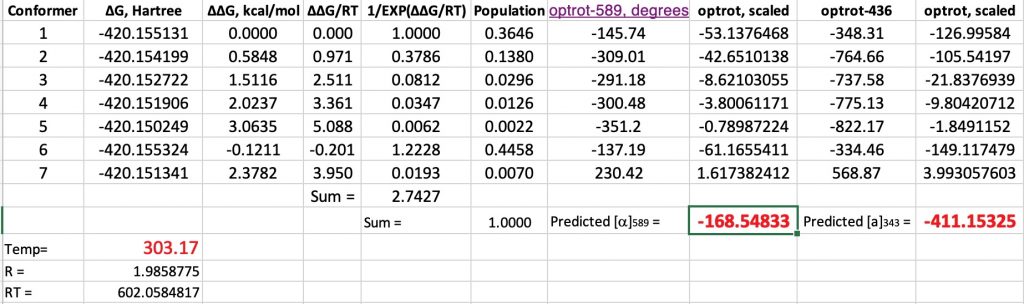
Here are the new values (FAIR data DOI: 10.14469/hpc/6445) obtained by adding an explicit water molecule to the original conformations. This also introduces extra conformations, some of which are included below. This reduces the calculated value by ~25° to improve the agreement with measurement (-147°). The value at 436nm is -411 (calc), -380 (obs).
So a 25° correction is not entirely insignificant, but does not change the overall conclusion that the optical rotation of D-(+) glyceraldehyde is (-). How might the model be improved further?
- Adding more water molecules, in theory until a limit is reached where further anisotropy is not added to the model. But the major disadvantage is that each extra water molecule increases the conformational space to be explored. With say seven added water molecules, there are probably 100s of conformations that would have to be searched, now a major undertaking.
- Another interesting avenue to explore is the temperature dependence of the optical rotation. The experimental values are shown below. This is due to the change in Boltzmann populations as a function of temperature.

At 343K, the original calculations without inclusion of a water molecule reduce the calculated rotation changes from -193 to -182.5, or about 10°. The observed value is a change of 39°. However, with the model including an extra water molecule, the value changes from -168.5 to -171°. So it might well be that reproduction of the temperature effects will require more water molecules added to the model.