A Test of the Single Conformation Hypothesis in the Analysis of NOESY Distance Data for Small Molecules

Abstract

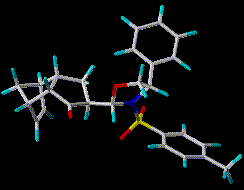Tricyclic ketone 1

1. Introduction
2. Methods
3. Results
4. Conclusions
5. References

Introduction

Multi-dimensional NMR has become a powerful complement to X-ray crystallography for the determination of molecular structure in solution. Among others, the three-dimensional architectures of proteins, oligonucleotides and macromolecular complexes have been convincingly established in aqueous media. [4] The methodology generally involves the measurement of a large number of geometrically determined variables such as nOe's and coupling constants (J's) followed by a search of the target structure in 3-space constrained by the same variables. For example, starting from a number of points, restrained molecular dynamics (MD) routinely leads to a common, internally consistent 3-D structure. [5] The tactics are successful, in part, because the macromolecules in question are relatively conformationally immobile. Apart from small subsets of disordered loops, the molecules present essentially a single target conformation.

Peptides or small flexible organic molecules show a fundamentally different conformational profile in solution across a range of temperatures. That is, the compounds are usually characterized by families of rapidly equilibrating conformations characterized by an average NMR spectrum. While there are a few approaches to deconvoluting the average, [6] it has become popular to apply the macromolecular techniques to small molecules in solvent under the assumption of a single important conformation. Structures reasonably consistent with NOESY, ROESY and coupling constant data are thereby derived and offered as a meaningful solution to the conformational problem. [7,8,9]

The present contribution examines this proposition for tricyclic ketone 1, an enantiomerically pure diastereomer synthesized by Diels-Alder cycloaddition in a study of asymmetric induction.[10] Compound 1 has been subjected by Reggelin and coworkers to a careful NOESY analysis in CDCl3 leading to 50 apparently well-determined intramolecular distances and one key J(H1-H6) = 2.9 Hz. [1] Restrained MD and the assumption of a strongly preferred conformation yielded a diastereomeric configuration and conformation similar to that for a closely related structure derived by X-ray crystallography. [11]

Methods

In the present work, conformational profiles of the endo and exo isomers of 1 were obtained by 1000 step Monte Carlo searches with the MM2* force field and the GB/SA CHCl3 solvent continuum model in MacroModel. [2] A total of 37 and 58 fully optimized conformations within 10 kcal/mol of the respective global minima resulted. The fact that the global minima were found 15 and 13 times, respectively, provides a high measure of confidence that a thorough search of the low energy regions of conformation space has been achieved.

A NAMFIS analysis was carried out for each of these data sets with the nOe determined distances and J(H1-H6).The recently disclosed method (NMR Analysis of Molecular Flexibility in Solution) [3] combines empirical and modeled structural variables by a non-linear least squares procedure to yield a "best fit" solution to the geometrically transformed 2-D NMR spectrum (or spectra). Goodness-of-fit is expressed as the sum of square differences (SSD) between measured and modeled variables. In practice, the procedure operates on a "complete" set of molecular conformations and deconvolutes the thermally averaged NMR variables into a small family of conformations that optimally represents the J-derived torsions and nOe-derived distances. The population of conformers (set of molar fractions) that best fits both the experimental NMR data and the associated errors is considered a "feasible solution". In the present case a separate error analysis has not been performed for each NMR distance and torsion. Instead, the following error estimates are assigned to the nOe distance categories below:

          error	    nOe distances (x in Å)
	+/- 0.1		       x < 2.5 
	    0.2		2.5 <= x < 3.0
	    0.3		3.0 <= x < 3.5
	    0.4		3.5 <= x < 6.0

The result of the fitting exercise is thus termed a "best solution". Roughly speaking, the outcome is 80% probable at the 95% confidence level.

Results

The exo dataset yielded a relatively poor four-conformation fit to the NMR data with SSD = 45. The outcome is consistent with the previous nOe/restrained MD examination which ruled out the exo isomer as the product of the Diels-Alder reaction. [1] By contrast, the endo dataset delivered a four-conformation fit with SSD = 9 and J(H1-H6) = 2.6 Hz (Jexp = 2.9 Hz). Most of this error is due to deviations in calculated and measured nOe distances involving the meta protons on the phenyl subunit.

Tables 1 and 2 display the predicted percentages of each conformer in the endo and exo sets, respectively, as well as the values of the J(H1-H6)-derived torsion. For comparison, the single restrained MD conformation of 1 was reconstituted from data in the Reggelin work by reoptimizing the structure (MM2*) under the constraint that both the torsional angles around C1-C6 and the intramolecular distances conform to the reported MD values (SSD = 3 when fit to MD values). However, NAMFIS fitting of the MD structure to the NMR data led to the unusually high SSD = 31 and J(H1-H6) = 3.5 Hz. The MM2* MD-structure was subsequently reoptimized without constraints leading to an energy drop of 16.4 kcal/mol. The structure is clearly distant from the nearest local minimum on the MM2* potential energy surface.

The MD structure obtained above (white in figure) was compared with the "best fit" family of endo conformers. Torsions describing the two rings (oxazolidine, cyclopentanone), the C1-C6 dihedral, and the torsions about N-S, S-Ctol, and C-Cph are listed in Tables 3 & 4 and Tables 5 & 6. Figure 3 illustrates the atomic numbering. The MD conformer is distinct from each of the conformers in the NAMFIS population. For comparison, the values for an average structure of the NAMFIS conformers (weighted by mole fraction) is also included in Tables 3 - 6. The average, a "virtual" conformer, is actually closer to the MD structure than any individual optimized conformer.

Conclusions

We conclude that tricyclic ketone 1 is not well-characterized by a single conformation in CDCl3 solution, but by a set of rapidly equilibrating conformers that produce a deceptively averaged NMR spectrum. It would appear that the previously suggested structure is a virtual conformation which combines multi-conformer features in a single geometry. As such, the probability of its existence on the conformational surface of the endo Diels-Alder adduct is negligible. In general, the presumption of single or strongly preferred conformation for a small molecule characterized by easily rotated bonds involves both a risk and a deep-seated assumption. The assertion is best tested by independent means prior to mapping NMR distances and torsions onto a single aggregate of atoms.

References

[1] Reggelin, M.; Hoffmann, H.; Kock, M.; Mierke, D.F. J. Am. Chem. Soc. 1992,114, 3272.

[2] Mohamadi, F.; Richards, N.G.J.; Guida, W.C.; Liskamp, R.; Lipton, M.; Caufield, C.; Chang, G.; Hendrickson, T.; Still W.C. J. Comp. Chem. 1990, 4, 440.

[3] Cicero, D.O.; Barbato, G.; Bazzo, R. J. Am. Chem. Soc. 1995,117, 1027.

[4] Wutrich, K.; NMR of Proteins and Nucleic Acids; Wiley: New York, 1986.

[5] Brunger, A. T.; Clore, G. M.; Gronenborn, A. M.; Karplus, M; Proc. Natl. Acad. Sci. U.S.A. 1986, 83, 3801; Kuszewski, J.; Nilges, M.; Brunger, A. T. J. Biomol. NMR, 1992, 2, 33; Powers, R; Garrett, D. S.; March, C. J.; Frieden, E. A.; Gronenborn, A. M.; Clore, G. M. Biochemistry, 1993, 32, 6744.

[6] Landis, C; Allured, V. S. J. Am. Chem. Soc., 1991, 113, 9493; Bruschweiler, R.; Blackledge, M.; Ernst, R. R. J. Biomol. NMR, 1991, 1, 3; Nikiforovich, G. V.; Prakash, O.; Gehrig, C. A.; Hruby, V. J. J. J. Am. Chem. Soc., 1993, 115, 3399.

[7] Cachau, R. E.; Gussio, R.; Beutler, J. A.; Chmurny, G. N.; Hilton, B. D.; Muschik, G. M.; Erickson, J. W. Supercomputer Applic. and High Performance Comput. 1994,8, 24.

[8] Paloma, L. G.; Guy, R. K.; Wrasidio, W.; Nicolaou, K. C. Chemistry and Biology 1994, 1, 107.

[9] Walker, S.; Valentine, K. G.; Kahne, D. J. Am. Chem. Soc., 1990, 112, 6428; Walker, S.; Yang, D.; Kahne, D. J. Am. Chem. Soc., 1991, 113, 4716; Walker, S.; Murnick, J.; Kahne, D. J. Am. Chem. Soc., 1993, 115, 7954.

[10] Hoffmann, H.; Bolte, M.; Berger, B.; Hoppe, D. Tetrahedron Lett. 1993, 6537.

[11] Citations 18 in reference [1].